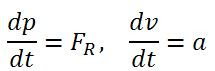Salen This!

You know the perfect gift for someone who likes shiny, yellow crystals? Well, watch this space and I'll teach you how! :p

-----------------------------

Enter salicylaldehyde, commonly referred to as salen, which is a very common ligand in Organometallic Chemistry:

And in its pure state, it manifests itself in the form of flaky, shiny yellow crystals! It's really intriguing, because one usually thinks of crystals as inorganic compounds, consisting of metal ions and their salts. Yet this is but an organic compound, and whenceforth the colour?

Only time will give the answer!

Now You Oxy-It, Now You Don't!

Alright, after a failed attempt at the retrosynthesis of haemoglobin, let's just marvel at nature's molecule:

Freaky. :p

Carboshift!

I remember reading in this article, where there was a study made on carbocations in solution; apparently they managed to stabilise the formation of carbocations in superacidic solution, and therefore observed the characteristics of carbocations, in particular their fluxional character. And they found that when they prepared the carbocation that had the structure:




After NMR analysis, they found that they had two other carbocations:



Can you fashion a guess? Haha.

------------------------------------------------

And if you still haven't figured it out yet, it's due to carbocationic rearrangements as such:


Quite cool huh? Haha.

Circular Argument I

Here's something that caught my attention when I was tutoring one of my students in 'E' Math at the Secondary School level: the angle subtended by a chord of a circle at the centre of the circle is twice that subtended by the same chord at the circumference.

Perhaps a diagram might make things much easier:


The chord is the line AB, and the angle subtended at the centre is the angle AOB, and the angle subtended at the circumference is the angle ACB, as indicated in Greek above, haha. So this property of a circle says that angle AOB is twice that of angle ACB. But why?

Easy enough, I shall use the method that Shaun used during our lecture, which is a rather neat and easy proof; so kudos to Shaun! First of all, you divide the triangle ABC into half, down the centre as shown below with a line CD, and then notice I've coloured an isoceles triangle in green:

And then you should notice that this line should bisect the angles AOB and ACB, and we can conclude first that:

And from this, the following statements should therefore make sense:


Quite a neat proof huh? Shaun came up with it all by himself, pro!

Speeding Towards Light

So, someone tagged that she wants to know why light always travels at the speed of light huh? Haha. Well here’s some information to get you started:

This is a typical wavefunction, which is a function of both position (x) and time (t). If you’re a good JC student in Singapore taking Mathematics at the H2 level, then you should be able to figure out that this function travels in the direction of positive x (if you can’t tell you can come ask me, heh) and it also travels in this direction with increasing time.

Now, we see that there’s an amplitude (A), and there’s an angular frequency (ω), and there’s a wavenumber (k). These quantities should, hopefully, be familiar to you, or at least I hope you’ve heard of them! Haha. These are all A Level knowledge, so hopefully you have!

I’d like to say that although what I’m about to say applies to all waves, not all waves have such a nice looking function like the one above! I just chose the easiest one of all so that it’s easy to digest what I’m going to say.

Going on, I’d like you to see that:


What I’ve done here is to take the second partial derivative, meaning I differentiate the wavefunction with respect to x only, twice, each time keeping t constant.

Aright, now, let’s try to take the partial derivative again, now with respect to t:


Cool! To sum it all up so that you follow me better:


It should be obvious to you then, that:


Does anyone want to fashion a guess what this means? Heh. Well, for waves we know that (from basic JC level Physics):


And therefore, we can say that:


But hey! We learnt in Secondary School that the speed v of any wave is related to the frequency f and wavelength λ by:


And therefore:


And thus our previous equation shows that:


That is, if we differentiate the wavefunction with respect to position twice, we obtain the derivative of the wavefunction with respect to time divided by the speed of the wave squared! What an interesting inherent symmetry! And this holds for all waves!

I’d really like to go on, but well, I don’t think most of you are acquainted with electromagnetic waves, are you? For electromagnetic waves, the equation turns out such that:


If you don’t know, ε is the permittivity of vacuum, and μ is the permeability of vacuum. Interesting enough, we note that:


Since all quantities on the left are constants, then we conclude that the speed of light (or rather, electromagnetic waves) must also be a constant regardless of anything, and therefore we now have:


Well well, I hope this is good enough! Because really, I can’t explain why light has a constant speed unless one deals with more advanced electromagnetic theory and wave mechanics. But haha, this is good enough I think, for all of you to chew upon! :p

Double Angle Madness III

And I might as well show the last identity, right? Haha, and so here it is:

And that's all for now. :)

Double Angle Madness II

And continuing with the streak, I might as well show how to prove a very commonly used identity; for convenience, I've reused the diagram from the previous post:



Trigonometry isn't that hard right? :)

Double Angle Madness

Well, I gave one of my tuition students this identity to prove:

sin 2x = 2 sinx cosx

And is it really that hard? Well, actually all it needs is a single diagram and two lines of working, as I have illustrated below:

Cheers! :)

Newton's Legacy

Here’s something for you to think about – Newton’s Second Law is often quoted as the net external force on an object is the product of the object’s mass and its acceleration. Well I wouldn’t say that’s wrong, but that’s only truly correct in a classical sense. That is, the following equation only holds for non-relativistic speeds:


The more accurate way to quote Newton’s Second Law, which holds for all situations, is this: the net external force on an object is the time rate of change of momentum of the object itself. And we express this mathematically as:


And I’ll illustrate the generality of this expression by using this to derive a relativistic expression for the acceleration of a body. Now, to start off, let us recall the relativistic momentum expression:


And therefore the rate of change of momentum of a body must be given by a direct differentiation with respect to time:


And if you simplify this, it becomes:


And we recognize two things, that is:


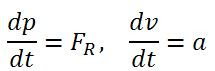
And therefore:


Notice that Newton’s Second Law no longer just involves the product of the mass and acceleration – this means that the expression F = ma is not a generally valid expression! And hence my conviction that Newton’s Second Law should be reformulated in terms of rate of momentum change instead.

Now, let us rearrange what we have above:


So let’s say you want to accelerate a body to the speed of light (c ms^-1), notice that as the speed v tends towards c, the acceleration tends to zero for a finite force:


So how do you accelerate something to a speed of c given that the acceleration fades away to zero when you near the speed of light? Easy, you use an infinite force! But is there such a thing as an infinite force? No! However, is there another way to go about it? Well, yes! Notice that as the mass goes towards zero:


Therefore, if the mass of a body is zero, then it is possible to accelerate the body all the way to the speed of light!

Ask yourself, what is the mass of a photon? :p It’s simply zero, which is expected! Otherwise it can’t go at the speed of light! :)

God's Thoughts

"I want to know how God created this world. I am not interested in this or that phenomenon. I want to know His thoughts, the rest are details." - Albert Einstein, Jewish Physicist

And this is what one of the greatest minds who ever lived on this Earth once said - and I find it so encouraging that even a giant like Albert Einstein was after God's heart and mind, and how his Creation came into place.

I'd like to emphasise that all Science around us, all Mathematics, is only but an attempt to describe and understand this world, which is based on the laws that God engineered for all of us. Seeing how this intricate clockwork has come together, His handiwork is truly in the firmaments. :)

*Note: Albert Einstein wasn't a Christian even though he pursued the thoughts of God.

At The Speed of Light

It's now 8:00 am in the morning, and I just had this flash of inspiration whilst in the toilet; not too glamourous a situation for a brainwave, but oh well. Hopefully you all can understand what I'm about to type out.

Many years ago, Einstein as a young lad was thinking to himself: "What would happen or what would I see if I travelled at the speed of light? Would I see light waves or photons freeze in their tracks because I'm moving just as fast as them and thus the relative velocity between them and me is zero?"

And many years later, Einstein was convinced that no matter how fast one travels at, even at the speed of light, one will still see light travel at the speed of light.

Why?

Well, to first understand the situation, you must first understand the fundamental problem in Einstein's gedanken, that is, can you even see a stationary light wave?

The answer is a resounding and definite NO! Light, being composed of photons, are massless particles. From Einstein's mass energy equation, we therefore know that the total energy of a photon must be composed of its momentum-energy, that is, its kinetic energy, because it has no mass at all.

Now, to view a photon that is stationary, is then to view a photon without its kinetic energy - by denying a photon its kinetic energy, one essentialy annihillates that photon from sight. By travelling up to the speed of light, and to insist that one can still see light, then the light waves that one sees while travelling at the speed of light, must still be moving, and can't be at zero velocity.

You all got that? Haha.

And I guess I'll stop here for a while - I'll come back to explain further (in another post) why you need to be massless to move at the speed of light. :)

Derivator!

Look at this second-order equation:

How would you solve it? I mean, there’s always the conventional method of assuming a solution that has an exponential form, but did you know that you can treat this like a quadratic equation?

The method is known as the solution of second order differential equations via factorization, which isn’t really an original method thought out by me, but well, it helps to explain why the solution is of an exponential form. First of all, we factorize out the y term:

Then of course, we make a simplification; that is we allow:

And therefore we must insist that:

Of course, let us factorize this further:

In which case we must therefore have:

And of course if we solve for these two equations, we have:

And of course, the general solution being the sum of the two particular solutions we obtained earlier, from the principle of superposition for linear equations:

And if you only want a real solution, then:

And this explains why we always assume the solution is of an exponential form; simply because if you do solve it from first principles, it always is! :)

Resonance Imaging

So, some of you think Resonance Theory is outdated huh? Well, try this question! Do you think you can tell me which carbon atoms would most likely bear the positive charge in this cation without Resonance Theory:

I've drawn out the resonance hybrid (i.e. the actual molecular structure) of this ion. I'll give you a hint: there are four carbon atoms that could bear the positive charge. :)

------------------------------------------------

It seems like no one has given a go at this, but oh very well; if you can mentally draw the set of resonance structures in your head, this should really be problem at all:

And that's that! Haha.

Summation Woes

Well, here's something that both JX and Luoning can understand and appreciate; it's basically something I discussed with Shaun over MSN, and it's an alternating series, taking the form of:


Notice that we can group the terms like this and obtain a sum for an odd number of terms:


Also, we can group the terms like this and obtain a sum for an even number of terms:


The strange thing is, notice that if you consider an even number of terms, the sum goes to positive infinity, and if you consider an odd number of terms, the sum goes to negative infinity! This is what we call an oscillating series, which happens to be diverging as well.

I’ve included a graph for your reference, to illustrate the oscillating and diverging nature of this series:


Notice the diverging nature of the sum to n terms, and consequently, there can be no sum to infinity, simply because the sum to n terms depends on the very number of terms, and thus on the last term. A sum to infinity where the number of terms and the last term is not defined can therefore produce no well defined result.